CLOCKS(Angle between minute hand and hour hand)

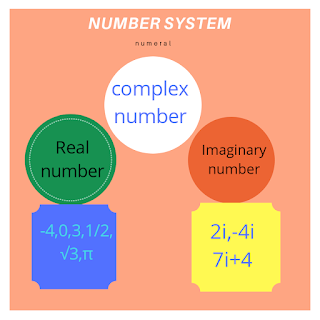
Important points-

Clock-1.A clock has two hands,the smaller one is called the hour hand or short hand while the longer one is called the minute hand or long hand.

2.The face or dial of a watch is a circle whose cicumference is divided into 60 equal parts,called minute space.

3.In every hour, both the hands concide once.

4.In 12 hours ,both the hands concide 11 times. 

5.In 24 hours,both hands concide 22 times.

6.When the two hands are at right angle,they are 15 minutes space apart.

6.When the two hands are in opposite directions, they are 30 minute space apart.

*Angle between minute hand and hour hand:-

1.Find the angle between minute hand and hour hand of the following figure.

Sol:-

2.Find the angle between minute hand and hour hand of the following figure.

Sol:-                                          

A DAY FOR ANY DATE

Step-
1.First we assign a code number to every day of the week. They are easy to remember.

2.Next we need a code for every month of the year.These month codes are used for every year,with two exceptions.

Etty

*In a leap year,the code for January is 5 and the code for February is 1.
3.Every year is assigned a code number.
Year of code-
1.There are 5 odd days in every 100 years.
2.There are 0 odd days in every 400 years.
Example-The code of 1900 .
1900=1600+300.
Code=0+5×3=15.(multiple of 400 is 0)
Example-The code of 1800.
1800=1600+200.
Code=0+5×2=10.(multiple of 400 is 0)
How to calculate day for date-
1.Day of date july4,1776.
a.code of  1776=1700+76.
1700=1600+100=0+5×1=5.
Code of 1776=5+76+76/4=5+76+19=100.
YEAR CODE+MONTH CODE+DATE=100+5+4
=109,it is divided by 7(because day code is 1 to 7) and take remainder.
Here remainder is 4.
Day code is Thursday.
So,July 4 ,1776 is Thursday.
Determine the days of the following dates:
1.June14, 2012
2.September1,1983.

Quadratic equation

Quadratic equation:-An equation of the form ax²+bx+c=0 where a,b and c ∈ R and a≠0 is called a quadratic equation.

Note-(1)An equation of degree 2 is called a quadratic equation.

Ex-1.x²+5x+6=0

      2.x²+7x+10=0

      3.x²-5x+10=0

      4.2x²+8x+6=0

Solution or roots of a quadratic equation:-  If p(x)=0 is a quadratic equation,then the zeros of the polynomial p(x) are called the solutions or roots of the quadratic equation p(x)=0.

Note:-1.Since the degree of a quadratic equation is 2,it has 2 roots or solutions.

2.x=a is the root of p(x)=0,if p(a)=0.

Method of solving a quadratic equation-

(a)spliting the middle term:-

1. x²+5x+6=0.

split the middle term 5x=3x+2x ,because multiplication of extreme terms(x²×6)=6x²=3x×2x.

Here x²+3x+2x+6=0

       ⇒ x(x+3)+2(x+3)=0

        ⇒(x+3)(x+2)=0

         ⇒x=-3 ,-2

2. x²-7x+12=0 

⇒x²-4x-3x+12=0, because multiplication of extreme term x²×12=(-4x)(-3x)=12x²

⇒x(x-4)-3(x-4)=0

⇒(x-4)(x-3)=0

⇒x=4, 3

3. 2x²+12x+16=0

⇒2x²+8x+4x+16=0

⇒2x(x+4)+4(x+4)=0

⇒(x+4)(2x+4)=0

⇒x=-4 ,-2

solve the following quadratic equation.

1.x²+5x+4=0

2.x²-5x+4=0

3.x²+5x-6=0

4.x²-5x-6=0

Properties of multiplication

Multiplication:-

1.Multiplication of any number by 11:-

   45×11=4 (4+5) 5=495

   25×11=2 (2+5) 5=275

   37×11=3 (3+7) 7=3(10)7=407(Add carry on      next digit).

    123×11=1 (1+2) (2+3) 3=1353.

    217×11=2 (2+1) (1+7) 7=2387.

    531×11=5 (5+3) (3+1) 1=5841.

    679×11=6 (6+7) (7+9) 9=6(13)(16)9=7469.
 
     ( Add carry on next digit).




      Follow on my blogs.
                                      

Square root

The square root of positive numbers-


1. square root(4)=+2, -2                                                                                                                        
   2.    √4=2                                                          
       symbol '√ 'this represet  only for     positive value.                                                                                                                                                       square root(4)≠√4 .                                 3. if x²=16 then x=4 , -4                                              if x=√16 then x=4.                                                                                                                      

Some important formulae

Formulae:-

1. 1+2 +3+4+...........n=n(n+1)/2.

Ex:-1+2+3+4+5+6+7+8=8(8+1)/2=36

2. 2+4+6+8+..........n terms=n(n+1)

Ex:-2+4+6+8+10+12+14=7(7+1)=56.

3. 1+3+5+7+..........n terms=n²

Ex-1+3+5+7+9+11+13+15+17=9²⁼81

Tricky method(square of numbers)

Square of numbers:-

 

    ( a)⇒        21²=20²+1(20+21)=441

                      22²=20²+2(20+22)=484

                      23²=20²+3(20+23)=529

    (b)⇒         29²=30²-1(29+30)=841

                      28²=30²-2(28+30)=784

   (c)⇒           71²=70²+1(70+71)=5041

                       72²=70²+2(70+72)=5184

   (d)⇒            79²=80²-1(79+80)=6241

                        78²=80²-2(78+80)=6084

 Note-This trick is valid for any digits number

Family of numbers

Family of numbers-

Complex number-It is denoted by "Z".

  Ex-0,-2,8,15/7,2i+8,√7.

Real number-It is denoted by "R".

 Ex-0,-5,27,3/8,√12.

Imaginary number-It is denoted by "I".

 Ex-2i,3+4i,-7i.

Note- i=√-1.

Properties of perfect square

Properties:-

1. Units digit of a perfect square can be-0(even number of zeroes),1,4,5,6,9.
Ex-100,81,144,225,676,289.
2. Digital sum of any perfect square can be-1,4,9,7.
Ex-289 digital sum=2+8+9=19,digital sum=10,digital sum=1.(until one digit). 
Note-perfect square cannot end with an odd number of zeroes and the digits2,3,7,8.

Divisibility rules(12)

Divisibility rule of 12:-                                        

12=4×3, HCF(3,4)=1

so,we say that a number which is divisible by 3 and 4 both, then the number will be divisible by 12.

Ex-108 is divisible by 3 and 4 ,then 108 will be divisible by 12.

Ex-216,3432,300,6000,1008.

  

                                   

Divisibility rules(11)

Divisibility rule of 11:-

 Number=abcdef

                sum of odd places digits=b+d+f.

                sum of even plces digits=a+c+e.

       Differnce of these two =(b+d+f)-(a+c+e)

       should be divisible by 11(0,11,22 ........ ).  

         Ex-8294,

          Sum of odd places digits=4+2=6.

          Sum of even places digits=9+8=17.

      Difference=17-6=11,here difference is divisible by 11,so 8294 is also divisible by 11.

                 

Divisibility rules(7)

1.Divisibility rule of 7:-


       

           161➝16-1×2=14, it is divisible by 7,                 
              so 161 will be divisible by 7.

             273⟶27-3྾2=21 it is divisible by 7,
              so 273 will be divisible by 7.


             581➝58-1×2=56 it is divisible by 7,
              so 581 will be divisible by 7. 



   * Which of following numbers are         divisible by 7.
1. 301  2. 378 3.462  4.4501  5.994



* Fill in the blank with suitable number.

a .23*     so it is divisible by 7.

b.56*9     so it is divisible by 7.

c.32*6    so it is divisible by 7.

d.35425* so it is divisible by 7.

Divisibility rules

  Divisibility (➗)-

1. Divisibility rule of 5: -A number which has a unit place 0 or 5, then the number will be divisible by 5.Ex-125,123450,2375.

Note-Unit place should be 0 or 5.

2.Divisibility rule of 10:- A number is divisible by 10 if unit place is '0'.

Ex:-10,1206780,980,25250.

*Which of following is divisible by 5 and 10 ?
1.2340            2.5235           3.73290        4.9835


*What should be added of the following numbers that it is divisible by 5.
1.22           a.3    b.7   c.9   d.5

2.39            a.6   b.3   c.7    d.1

3.41             a.3   b.5   c.4    d.9

4.77             a.13  b.12  c.2   d.7

5.121            a.4    b.0     c.7   d.1

Divisibility rules of numbers

1. Divisibility rule of 3: -A number whose sum of digits is divisible by 3, then the number will be divisible by 3.                                                                                                                     
Ex:- 123, the sum of digits=1+2+3=6, divisible by 3, then 123 is also divisible by 3.

2. Divisibility rule of 6: -A number is divisible by 2 and 3 both, then the number is also divisible by 6.
Ex-23142 is divisible by 2 and 3, so 23142 is also divisible by 6.
Note-6=2★3, HCF(2,3)=1.

3. Divisibility rule of 9: -A number whose sum of digits is divisible by 9, then the number will be divisible by 9.

Ex:-342, the sum of digits=3+4+2=9, Divisible by 9, then 342 is also divisible by 9.

Divisibility rules of numbers

Divisibility rules-

1. Divisibility rule of 2 -A number whose unit place is divisible by 2 (unit place 0,2,4_6,8) then the number will be divisible by 2.Ex-124, here unit place '4' is divisible by 2 then 124 is also divisible by 2.

2. Divisibility rule of 4 - A number whose last two digits(number formed by unit place and ten place) is divisible by 4 then the number will be divisible by 4.Ex-432, here last two digits '32' is divisible by 4 then 432 is also divisible by 4.

3.Divisibility rule of 8-A number whose last three digits (number formed by unit place, ten place, and hundred place) is divisible by 8 then the number is also divisible by 8.     Ex-3456, here last three digits 456 is divisible by 8 then 3456 is also divisible by 8.

Note:- 2= 2¹→last one digit.

           4=2²→last two digits.

           8=2³→last three digits.

           

Properties of numbers

1. If two digits number and a number obtained by reversing its digits are added then it will be divisible by 11.

     Ex-12+21=33,it is divisible by 11.
     
           45+54=99, it is divisible by 11.

       2. If two digits number and a number obtained by reversing its digits are subtracted then it will be divisible by 9.

      Ex-65-56=9, it is divisible by 9.

           93-39=54, it is divisible by 9.

        3. If two digits number and its sum of digits are subtracted then it will be divisible by 9.
      Ex-13-(1+3)=9, it is divisible by 9.

           29-(2+9)=18, it is divisible by 9.

Pythagoras triplet

Pythagoras-

Born-570 BCE, Samos(Greece).
Died-500-490 BCE, Metapontum(Italy).
He was a Greek philosopher, mathematician. Pythagoras is most famous for his concept of geometry.

Pythagoras

          a²+b²=c²

        pythagoras theorem


  





Pythagoras triplet theorem:-Three natural numbers a, b and c are such that  a²+b²=c², Then a, b and c are called Pythagoras triplet.

                                      Ex-(3,4,5)
            
                                      3²+4²=5²
                                      Ex- (5,12,13)

                                      5²+12²=13²

General form-     2m, m²-1, and m²+1

Properties of odd numbers or even numbers

1.The product of two consecutive even numbers-

                    8×10=9²-1

         

                    12×14=13²-1

    

                    14×16=15²-1

                    16×18=17²-1

      

                    ......................

                   106×108=107²-1

      note-    (middle term)²-1

The great mathematician and astronomer.

Aryabhata was an extraordinary teacher and scholar.

He was a famous Indian mathematician and astronomer, born in a place called Taregna, in Bihar which literally means songs of stars in Bihari, is a small place situated near Patna.

                   Aryabhata(476-550A.D.)

Works of Aryabhata-

1.value of pi.  2. He discussed the idea of sin.

3. Describes the solar and lunar eclipses scientifically 4.invention of zero.

Properties of '0'

Properties of zero(0):-

1. If any number is multiplied by zero then becomes zero.
Ex- 0×5=0
       0×8=0
       0×45=0
2.If zero is added any numbers then numbers remain unchanged.
Ex- 0+2=2
       0+6=6
       0+50=50
3.If any number is divided by Zero then it becomes undefined.
            Ex-5/0,14/0(undefined).
4.If zero is divided by any numbers then it becomes zero.Ex-0/9=0,0/45=0

Properties of even numbers and odd numbers

properties 1. Odd numbers

→ 1+3=2²=4               (number of terms 2)

     1+3+5=3²=9           (number of terms 3)

     1+3+5+.........=n²     (number of terms n)
 
  properties 2.Even numbers

    →2+4=2(2+1)=6            (number of terms 2)

      2+4+6=3(3+1)=12       (number of terms 3)

    2+4+6+...........=n(n+1)  (number of terms n)


Properties 3.

          →   1+2+1=2²=4           (middle term 2)

               1+2+3+2+1=3²=9     (middle term 3)

          1+2+3+4+3+2+1=4²=16 (middle term 4)
 1+2+3.....+n+..........+3+2+1=n² (middle term n)

properties 4.

→         1²=1    (one digit)

            11²=121  (two digits)

         111²=12321 (three digits)

     1111²=1234321 (four digits)

Even numbers and odd numbers

EVEN NUMBER:-An integer which is completely divisible by '2'(unit place should be 0,2,4,6,8) is called an even number.Ex-0,4,6,8,10,1982,-16,-1470............
ODD NUMBER:-An integer which is not completely divisible by '2'(unit place should be 1,3,5,7,9) is called an odd number.Ex- 1,3,5,7,9,11,-25,-123,1427........

Important properties-

1. Difference between any two consecutive even or odd numbers is 2.
2. Sum of even numbers is an even number.ex- 4+8=12, 24+12+8=44.
3. Sum of any two odd numbers is an even number.ex- 1+3=4,7+9=16,11+(-3)=8.
4. Sum of an even number and an odd number is an odd number.ex-1+2=3,7+22=29,15+(-8)=7.